Topic relation between perimeter and area: The relation between perimeter and area is a fascinating topic in geometry that reveals how these two fundamental properties of shapes are interconnected. This article delves into the mathematical and practical aspects of this relationship, offering insights and examples that illustrate the importance and applications of understanding perimeter and area together.
Table of Content
- Relation Between Perimeter and Area
- Introduction to Perimeter and Area
- Basic Definitions and Formulas
- Perimeter and Area of Common Shapes
- Square and Rectangle
- Triangle
- Circle
- Polygon
- Understanding the Relationship Between Perimeter and Area
- Mathematical Relationship
- Geometric Interpretation
- Applications and Examples
- Real-world Applications
- Problems and Solutions
- Optimizing Perimeter and Area
- Maximizing Area with Fixed Perimeter
- Minimizing Perimeter with Fixed Area
- Advanced Topics
- Isoperimetric Inequality
- YOUTUBE: Xem video này để hiểu rõ hơn về mối quan hệ giữa chu vi và diện tích trong toán học và các ứng dụng thực tế.
Relation Between Perimeter and Area
The concepts of perimeter and area are fundamental in geometry and are closely related. Understanding their relationship helps in solving various mathematical problems involving different shapes.
Definitions
- Perimeter: The perimeter of a two-dimensional shape is the total distance around the shape. It is measured in linear units such as meters, centimeters, or inches.
- Area: The area of a two-dimensional shape is the measure of the space enclosed within the shape. It is measured in square units such as square meters, square centimeters, or square inches.
Formulas for Common Shapes
| Shape | Perimeter | Area |
|---|---|---|
| Square | \(4a\) | \(a^2\) |
| Rectangle | \(2(l + w)\) | \(lw\) |
| Triangle | \(a + b + c\) | \(\frac{1}{2} \times \text{base} \times \text{height}\) |
| Circle | \(2\pi r\) | \(\pi r^2\) |
Relationship Between Perimeter and Area
While the perimeter and area of a shape are related, they measure different aspects. Changing the dimensions of a shape while keeping the perimeter constant can lead to different areas, and vice versa. Here are some key points:
- Shapes with the same perimeter can have different areas. For example, a square and a rectangle with the same perimeter will have different areas.
- Shapes with the same area can have different perimeters. For example, a long, thin rectangle and a square can have the same area but different perimeters.
- The relationship between perimeter and area becomes more complex with irregular shapes.
Examples
- For a square with a side length of 6 units:
- Perimeter = \(4 \times 6 = 24\) units
- Area = \(6^2 = 36\) square units
- For a rectangle with a length of 6 units and width of 4 units:
- Perimeter = \(2 \times (6 + 4) = 20\) units
- Area = \(6 \times 4 = 24\) square units
Understanding the Relationship
To better understand the relationship between perimeter and area, consider the following exercise:
- Draw several rectangles with the same perimeter but different dimensions.
- Calculate the area for each rectangle. Notice how the area changes while the perimeter remains constant.
This exercise demonstrates that the relationship between perimeter and area depends on the specific dimensions of the shape.
In summary, while perimeter and area are related, they are distinct properties of shapes. Mastery of both concepts is essential for solving a wide range of geometric problems.

READ MORE:
Introduction to Perimeter and Area
Perimeter and area are fundamental concepts in geometry, each describing different aspects of a shape. Understanding these properties is essential for various applications in mathematics, science, engineering, and everyday life.
Perimeter is the total distance around the boundary of a two-dimensional shape. It is measured by summing the lengths of all sides of the shape. For example, the perimeter of a rectangle with length \( l \) and width \( w \) is given by:
\[ P = 2l + 2w \]
Area is the measure of the space enclosed within a shape. It is calculated based on the shape's dimensions. For a rectangle, the area is given by:
\[ A = l \times w \]
Let's explore the perimeter and area of some common shapes:
- Square: For a square with side length \( s \):
- Perimeter: \[ P = 4s \]
- Area: \[ A = s^2 \]
- Triangle: For a triangle with sides \( a \), \( b \), and \( c \):
- Perimeter: \[ P = a + b + c \]
- Area (using Heron's formula): \[ A = \sqrt{s(s-a)(s-b)(s-c)} \] where \( s = \frac{a+b+c}{2} \)
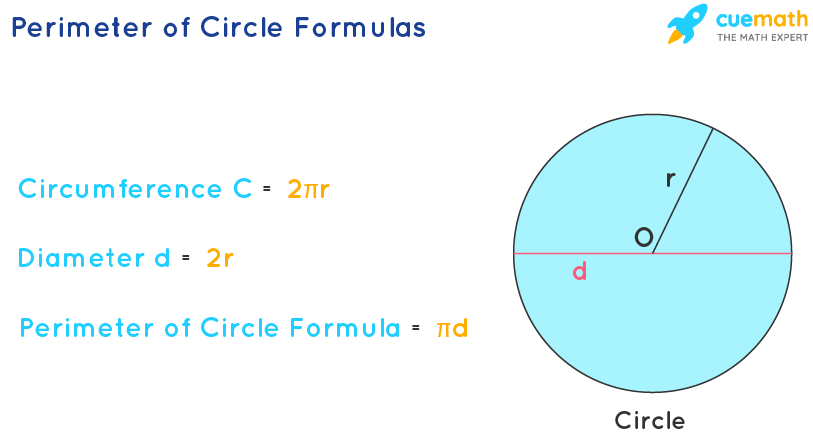
- Circle: For a circle with radius \( r \):
- Circumference (perimeter): \[ P = 2\pi r \]
- Area: \[ A = \pi r^2 \]
Understanding the relationship between perimeter and area helps in solving various geometric problems and optimizing designs in engineering and architecture. In the following sections, we will delve deeper into this relationship, exploring its mathematical foundations and practical applications.
Basic Definitions and Formulas
Understanding the basic definitions and formulas for perimeter and area is essential for grasping their relationship. Here, we provide step-by-step explanations for common shapes.
Rectangle
A rectangle has opposite sides of equal length. The perimeter and area are calculated as follows:
- Perimeter (P): The total distance around the rectangle. \[ P = 2l + 2w \] where \( l \) is the length and \( w \) is the width.
- Area (A): The space enclosed within the rectangle. \[ A = l \times w \]
Square
A square is a special type of rectangle where all sides are equal. The formulas are simplified as follows:
- Perimeter (P): \[ P = 4s \] where \( s \) is the side length.
- Area (A): \[ A = s^2 \]
Triangle
A triangle has three sides and its perimeter is the sum of its sides. The area can be calculated using different methods depending on the type of triangle:
- Perimeter (P): \[ P = a + b + c \] where \( a \), \( b \), and \( c \) are the lengths of the sides.
- Area (A): Using Heron's formula: \[ s = \frac{a+b+c}{2} \] \[ A = \sqrt{s(s-a)(s-b)(s-c)} \]
Circle
A circle is defined by its radius \( r \), with the following formulas:
- Circumference (C): The distance around the circle. \[ C = 2\pi r \]
- Area (A): The space enclosed by the circle. \[ A = \pi r^2 \]
Polygon
For a regular polygon (a polygon with all sides and angles equal), the perimeter and area can be calculated if the number of sides \( n \) and the length of a side \( s \) are known:
- Perimeter (P): \[ P = n \times s \]
- Area (A): Using the apothem \( a \) (a line from the center to the midpoint of a side): \[ A = \frac{1}{2} P \times a \]
These fundamental formulas are the building blocks for understanding the deeper relationship between perimeter and area, which we will explore in subsequent sections.
Perimeter and Area of Common Shapes
Understanding the relationship between perimeter and area involves exploring the characteristics of common geometric shapes. Here's a comprehensive guide:
- Square:
- Perimeter (P) = 4 * side length (s)
- Area (A) = side length (s) * side length (s) = s^2
- Rectangle:
- Perimeter (P) = 2 * (length + width)
- Area (A) = length * width
- Triangle:
- Perimeter (P) = sum of all three sides (a + b + c)
- Area (A) = (base * height) / 2
- Circle:
- Perimeter (Circumference) (P) = 2 * π * radius (r)
- Area (A) = π * radius (r)^2
- Polygon:
- Perimeter (P) = sum of lengths of all sides
- Area (A) = can be calculated using various methods depending on the type of polygon
These formulas provide a basis for understanding how changes in perimeter affect the area and vice versa, laying the groundwork for exploring the deeper relationship between these two fundamental geometric properties.
Square and Rectangle
The relationship between perimeter and area in squares and rectangles is fundamental to understanding geometric properties. Here's a detailed exploration:
- Square:
- Perimeter (P) = 4 * side length (s)
- Area (A) = side length (s) * side length (s) = s^2
- Rectangle:
- Perimeter (P) = 2 * (length + width)
- Area (A) = length * width
These formulas illustrate how changes in the dimensions of squares and rectangles affect both their perimeter and area. Understanding this relationship is crucial in various fields, from architecture to engineering, where optimizing space and efficiency is paramount.

Triangle
The relationship between perimeter and area in triangles is essential for understanding the geometry of this versatile shape. Here's a breakdown:
- Perimeter:
- To find the perimeter (P) of a triangle, add the lengths of all three sides (a + b + c).
- Area:
- To calculate the area (A) of a triangle, use the formula: A = (base * height) / 2.
These formulas demonstrate how changes in the sides and height of a triangle affect both its perimeter and area. Understanding this relationship is crucial in various applications, from architecture to trigonometry, where triangles are prevalent.
Circle
The relationship between perimeter and area in circles is fundamental to understanding the geometry of this curved shape. Here's a detailed explanation:
- Perimeter (Circumference):
- To find the perimeter (C) of a circle, use the formula: C = 2 * π * radius (r).
- Area:
- To calculate the area (A) of a circle, use the formula: A = π * radius (r)^2.
These formulas illustrate how changes in the radius of a circle affect both its perimeter (circumference) and area. Understanding this relationship is essential in various fields, from mathematics to engineering, where circles are prevalent in designs and calculations.
Polygon
The relationship between perimeter and area in polygons, which are multi-sided shapes, is key to understanding their geometric properties. Let's delve into the details:
For polygons with n sides:
- Perimeter:
- To find the perimeter (P) of a polygon, add the lengths of all its sides.
- Area:
- The area (A) of a polygon can be calculated using various methods, depending on its type and characteristics. Some common methods include:
- Using the formula for the area of regular polygons: A = (1/4) * n * side length^2 * cot(π/n), where n is the number of sides and cot represents the cotangent function.
- Dividing the polygon into triangles and summing their areas.
- Using the shoelace formula for finding the area of a polygon given the coordinates of its vertices.
Understanding how changes in the side lengths and angles of polygons affect their perimeter and area is crucial in various fields, including architecture, design, and surveying, where polygons are commonly encountered.
Understanding the Relationship Between Perimeter and Area
The relationship between perimeter and area is a fundamental concept in geometry, offering insights into the spatial properties of various shapes. Here's a detailed exploration:
- Basic Definitions:
- Perimeter: The perimeter of a shape is the total length of its boundary.
- Area: The area of a shape is the measure of the space enclosed by its boundary.
- Mathematical Relationship:
- While perimeter represents the length of the boundary, area signifies the extent of space enclosed within the boundary.
- Generally, increasing the perimeter of a shape does not necessarily result in an increase in its area, and vice versa.
- Geometric Interpretation:
- Understanding the relationship between perimeter and area involves visualizing how changes in the dimensions of a shape impact both its perimeter and area.
- For example, elongating the sides of a rectangle increases its perimeter but may not affect its area unless the dimensions are adjusted proportionately.
- Practical Implications:
- Knowledge of the relationship between perimeter and area is crucial in various fields such as architecture, engineering, and urban planning.
- Optimizing the perimeter and area of structures and layouts is essential for efficient space utilization and resource management.
By grasping the interplay between perimeter and area, one can gain a deeper understanding of geometric concepts and their practical applications.
Mathematical Relationship
The mathematical relationship between perimeter and area elucidates the intricate connection between the boundary and the space enclosed by geometric shapes. Here's a breakdown:
- Perimeter:
- The perimeter (P) of a shape is the sum of the lengths of its sides or the distance around its boundary.
- Area:
- The area (A) of a shape is the measure of the space enclosed within its boundary.
- Relationship:
- While perimeter focuses on the length of the boundary, area emphasizes the extent of space enclosed within the boundary.
- Increasing the perimeter of a shape does not necessarily result in an increase in its area, and vice versa.
- However, certain relationships exist between perimeter and area for specific shapes, such as squares, rectangles, triangles, and circles, where changes in dimensions affect both perimeter and area differently.
Understanding this mathematical relationship provides valuable insights into the spatial characteristics of geometric shapes and aids in various applications, including design, construction, and problem-solving in mathematics and engineering.
Geometric Interpretation
The geometric interpretation of the relationship between perimeter and area provides visual insights into how changes in the dimensions of shapes affect their boundary and enclosed space. Here's a detailed analysis:
- Visual Representation:
- Imagine a square with sides of length 1 unit. Its perimeter is 4 units, and its area is 1 square unit.
- Now, if we increase the length of each side to 2 units, the perimeter becomes 8 units, doubling the original perimeter. However, the area increases fourfold to 4 square units.
- This illustrates that while increasing the perimeter by a factor of 2, the area increases by a factor of 4.
- Shape Dynamics:
- For shapes like rectangles, elongating one side while keeping the other constant increases the perimeter without affecting the area, until adjustments are made proportionately.
- In contrast, for circles, increasing the radius enlarges both the perimeter (circumference) and the area, demonstrating a different relationship compared to polygons.
- Practical Implications:
- Understanding the geometric interpretation of the relationship between perimeter and area aids in designing efficient layouts, optimizing space utilization, and solving real-world problems in various fields such as architecture, urban planning, and landscaping.
- It also forms the basis for mathematical modeling and analysis in engineering and scientific research.
By visually interpreting the geometric properties of shapes, one can gain a deeper understanding of how changes in dimensions impact both perimeter and area, leading to more informed decision-making in practical applications.
Applications and Examples
The relationship between perimeter and area finds application in various real-world scenarios, influencing design, optimization, and problem-solving. Here are some applications and examples:
- Architecture and Construction:
- Architects use the understanding of perimeter and area to design buildings and structures efficiently, optimizing space utilization while considering aesthetic and functional aspects.
- For example, in designing floor plans, architects balance the perimeter to ensure structural integrity while maximizing interior space.
- Landscaping and Gardening:
- Landscape designers utilize the relationship between perimeter and area to plan garden layouts, determining the dimensions of flower beds, lawns, and pathways.
- By considering the area of different sections and the perimeter of pathways, designers create visually appealing and functional outdoor spaces.
- Engineering and Manufacturing:
- In engineering and manufacturing, knowledge of perimeter and area is crucial for optimizing material usage and product design.
- Engineers use geometric principles to design efficient structures, machinery, and components, minimizing material wastage and production costs.
- City Planning and Infrastructure:
- Urban planners consider the relationship between perimeter and area when designing road networks, parks, and public spaces.
- By analyzing the area and perimeter of different zones, planners ensure efficient land use and accessibility while enhancing the quality of urban life.
These examples demonstrate how understanding the relationship between perimeter and area enables professionals to make informed decisions and solve complex problems across diverse fields.
Real-world Applications
The relationship between perimeter and area has numerous practical applications across various fields, contributing to problem-solving and optimization in real-world scenarios. Let's explore some examples:
- Construction and Carpentry:
- In construction and carpentry, understanding perimeter and area is essential for accurate measurements and material estimation.
- Carpenters use the relationship between perimeter and area to calculate the amount of materials needed for projects such as flooring, tiling, and paneling.
- Finance and Economics:
- In finance and economics, the concept of perimeter and area is applied in risk management and investment analysis.
- For instance, financial analysts calculate the perimeter (risk) and area (return) of investment portfolios to assess their performance and make strategic decisions.
- Environmental Science:
- Environmental scientists use the relationship between perimeter and area to study habitat fragmentation and biodiversity conservation.
- By analyzing the perimeter-to-area ratio of fragmented habitats, researchers assess the impact on species dispersal and ecosystem resilience.
- Healthcare and Medicine:
- In healthcare and medicine, perimeter and area calculations are employed in medical imaging and diagnostic procedures.
- Physicians utilize the relationship between perimeter and area to measure and analyze anatomical structures and pathological lesions.
These real-world applications highlight the versatility and significance of the relationship between perimeter and area in diverse fields, demonstrating its relevance in problem-solving and decision-making processes.
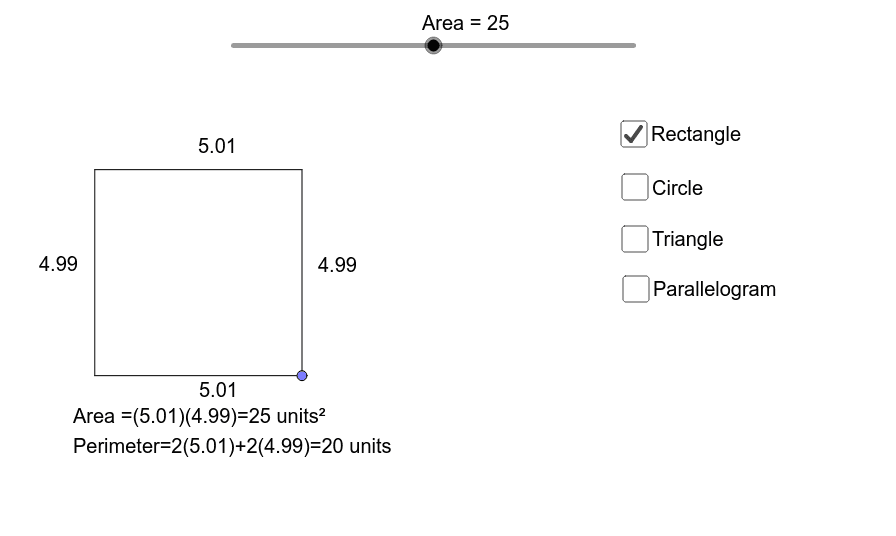
Problems and Solutions
This section presents a variety of problems and solutions related to the relationship between perimeter and area. Each problem is followed by a detailed solution to enhance understanding.
Problem 1: Rectangle with Fixed Perimeter
Problem: Given a rectangle with a fixed perimeter of 24 units, find the dimensions that maximize the area.
Solution:
- Let the length of the rectangle be \( l \) and the width be \( w \).
- Using the perimeter formula \( P = 2l + 2w \), we have:
- \( 2l + 2w = 24 \)
- \( l + w = 12 \)
- \( w = 12 - l \)
- The area \( A \) of the rectangle is given by \( A = l \times w \):
- \( A = l \times (12 - l) \)
- \( A = 12l - l^2 \)
- To maximize the area, take the derivative of \( A \) with respect to \( l \) and set it to zero:
- \( \frac{dA}{dl} = 12 - 2l = 0 \)
- \( 2l = 12 \)
- \( l = 6 \)
- Substitute \( l = 6 \) back into the equation for \( w \):
- \( w = 12 - 6 = 6 \)
- Therefore, the dimensions that maximize the area are \( 6 \) units by \( 6 \) units, and the maximum area is \( 36 \) square units.
Problem 2: Square with Fixed Area
Problem: Given a square with an area of 49 square units, find the perimeter.
Solution:
- Let the side length of the square be \( s \).
- Using the area formula \( A = s^2 \), we have:
- \( s^2 = 49 \)
- \( s = \sqrt{49} \)
- \( s = 7 \) units
- The perimeter \( P \) of the square is given by \( P = 4s \):
- \( P = 4 \times 7 = 28 \) units
- Therefore, the perimeter of the square is \( 28 \) units.
Problem 3: Circle with Given Perimeter (Circumference)
Problem: A circle has a circumference of 31.4 units. Find its radius and area.
Solution:
- Let the radius of the circle be \( r \).
- Using the circumference formula \( C = 2\pi r \), we have:
- \( 2\pi r = 31.4 \)
- \( r = \frac{31.4}{2\pi} \)
- \( r \approx \frac{31.4}{6.28} \)
- \( r \approx 5 \) units
- The area \( A \) of the circle is given by \( A = \pi r^2 \):
- \( A = \pi \times 5^2 \)
- \( A = \pi \times 25 \)
- \( A \approx 78.5 \) square units
- Therefore, the radius of the circle is approximately \( 5 \) units, and the area is approximately \( 78.5 \) square units.
Problem 4: Triangle with Fixed Perimeter
Problem: A triangle has a perimeter of 30 units. If two sides are 8 units and 12 units, find the third side and the area.
Solution:
- Let the third side of the triangle be \( c \).
- Using the perimeter formula \( P = a + b + c \), we have:
- \( 8 + 12 + c = 30 \)
- \( 20 + c = 30 \)
- \( c = 10 \) units
- Using Heron's formula to find the area:
- First, find the semi-perimeter \( s \):
- \( s = \frac{P}{2} = \frac{30}{2} = 15 \) units
- Then, the area \( A \) is:
- \( A = \sqrt{s(s-a)(s-b)(s-c)} \)
- \( A = \sqrt{15(15-8)(15-12)(15-10)} \)
- \( A = \sqrt{15 \times 7 \times 3 \times 5} \)
- \( A = \sqrt{1575} \)
- \( A \approx 39.68 \) square units
- First, find the semi-perimeter \( s \):
- Therefore, the third side of the triangle is \( 10 \) units, and the area is approximately \( 39.68 \) square units.
Optimizing Perimeter and Area
Understanding the relationship between perimeter and area is essential for solving various optimization problems. Here, we explore techniques to maximize area with a fixed perimeter and minimize perimeter with a fixed area.
Maximizing Area with Fixed Perimeter
To maximize the area of a shape with a given perimeter, regular shapes often provide the best solution. For instance, among all rectangles with a given perimeter, the square has the largest area. This can be demonstrated as follows:
- Consider a rectangle with length \( l \) and width \( w \).
- The perimeter \( P \) is given by: \[ P = 2l + 2w \]
- Solving for \( w \) in terms of \( l \) and \( P \): \[ w = \frac{P}{2} - l \]
- The area \( A \) of the rectangle is: \[ A = l \cdot w = l \left( \frac{P}{2} - l \right) = \frac{P}{2}l - l^2 \]
- To find the maximum area, take the derivative of \( A \) with respect to \( l \) and set it to zero: \[ \frac{dA}{dl} = \frac{P}{2} - 2l = 0 \Rightarrow l = \frac{P}{4} \]
- Thus, \( w \) is also \( \frac{P}{4} \), making the shape a square. The maximum area is then: \[ A = \left( \frac{P}{4} \right)^2 = \frac{P^2}{16} \]
Minimizing Perimeter with Fixed Area
To minimize the perimeter of a shape with a given area, the circle provides the best solution. Here’s why:
- Consider a shape with a fixed area \( A \).
- For a circle, the area \( A \) is: \[ A = \pi r^2 \]
- Solving for the radius \( r \): \[ r = \sqrt{\frac{A}{\pi}} \]
- The perimeter \( P \) (circumference) of the circle is: \[ P = 2\pi r = 2\pi \sqrt{\frac{A}{\pi}} = 2\sqrt{\pi A} \]
- This perimeter is less than that of any other shape with the same area. For comparison, consider a square with the same area: \[ \text{Side length} = \sqrt{A} \] \[ \text{Perimeter} = 4\sqrt{A} \] The square’s perimeter is larger than that of the circle for the same area.
Examples and Applications
- Example 1: A gardener wants to create the largest possible rectangular garden with 40 meters of fencing. The optimal shape is a square with each side measuring 10 meters, providing an area of 100 square meters.
- Example 2: An engineer needs to design a storage tank with a fixed volume but wants to use the least amount of material for the walls. A cylindrical shape (with a circular base) is often chosen for this reason.
Conclusion
By leveraging the mathematical relationships between perimeter and area, we can solve various optimization problems effectively. Regular shapes like squares and circles often provide optimal solutions for maximizing area with a fixed perimeter and minimizing perimeter with a fixed area.
Maximizing Area with Fixed Perimeter
When dealing with shapes with a fixed perimeter, such as a rectangle or a circle, maximizing the area becomes a crucial problem in various real-world applications, ranging from fencing a garden to designing efficient packaging.
To maximize the area enclosed by a shape with a fixed perimeter, we need to find the shape that utilizes the given perimeter most efficiently.
Let's consider the case of a rectangle with a fixed perimeter, where the perimeter is represented by the sum of all its sides: \(P = 2l + 2w\), where \(l\) is the length and \(w\) is the width.
To maximize the area (\(A = lw\)) of the rectangle, we can use calculus by expressing the area in terms of one variable. Since the perimeter is fixed, we can rewrite the equation for the perimeter in terms of one variable. For example, we can express \(l\) in terms of \(w\) using \(l = \frac{P}{2} - w\).
Now, substituting this expression for \(l\) into the equation for area, we get \(A = w(\frac{P}{2} - w)\).
To find the maximum area, we can take the derivative of \(A\) with respect to \(w\), set it equal to zero, and solve for \(w\).
Similarly, for a circle with a fixed perimeter, which is given by \(P = 2\pi r\), where \(r\) is the radius, we can maximize the area (\(A = \pi r^2\)) by using calculus techniques.
By finding the critical points of the area function and evaluating them, we can determine the radius that maximizes the area for a given perimeter.
In summary, maximizing the area with a fixed perimeter involves using optimization techniques from calculus to find the dimensions that yield the largest possible area for a given perimeter.
Minimizing Perimeter with Fixed Area
When confronted with the task of enclosing a fixed area with the least amount of material, minimizing the perimeter becomes a critical consideration in various practical scenarios, such as constructing fences or designing compact structures.
To minimize the perimeter while keeping the area fixed, we need to determine the shape that can enclose the given area with the shortest perimeter possible.
Let's explore this concept with the example of a circle, where the area (\(A = \pi r^2\)) is fixed and we aim to minimize the perimeter (\(P = 2\pi r\)).
Using calculus techniques, we can express the perimeter in terms of one variable. Since the area is fixed, we can rewrite the equation for area in terms of one variable. For a circle, the radius \(r\) can be expressed as \(r = \sqrt{\frac{A}{\pi}}\).
Substituting this expression for \(r\) into the equation for perimeter, we get \(P = 2\pi \sqrt{\frac{A}{\pi}}\).
To minimize the perimeter, we can take the derivative of \(P\) with respect to \(A\), set it equal to zero, and solve for \(A\).
Similarly, for other shapes such as rectangles or polygons with a fixed area, we can apply similar optimization techniques to find the dimensions that result in the smallest perimeter.
In summary, minimizing the perimeter with a fixed area involves using optimization methods from calculus to determine the dimensions that require the least amount of material to enclose the given area.

Advanced Topics
Exploring the relationship between perimeter and area leads us to delve into several advanced topics that are of significant interest in mathematics and various fields of application.
- Isoperimetric Inequality: This theorem states that among all closed curves with a given perimeter, the circle has the largest area. It has profound implications in geometry, optimization, and mathematical physics.
- Perimeter and Area in Complex Shapes: Analyzing the perimeter and area of irregular or complex shapes involves advanced geometric techniques such as decomposition, approximation, and integration. Understanding these concepts is crucial in fields like architecture, computer graphics, and urban planning.
- Perimeter-Area Calculations in Various Fields: The relationship between perimeter and area finds applications in diverse fields such as biology (studying cell membranes), economics (maximizing profit margins), and ecology (analyzing habitat fragmentation). Exploring these applications enhances our understanding of the interplay between geometry and real-world phenomena.
By delving into these advanced topics, we gain deeper insights into the intricate connections between perimeter and area, uncovering their significance across different domains of knowledge and application.
Isoperimetric Inequality
The isoperimetric inequality is a fundamental result in geometry that establishes a relationship between the perimeter and area of a closed plane curve or shape.
It states that among all closed curves with the same perimeter, the circle encloses the maximum area. In other words, if we have a fixed length of material to create a closed shape, the circle will enclose the largest area compared to any other shape.
This inequality has profound implications in various areas of mathematics, physics, and engineering. It is often used as a basis for optimization problems where the goal is to maximize or minimize certain quantities subject to constraints.
The isoperimetric inequality has been studied extensively since antiquity and has inspired numerous mathematical investigations and proofs. It serves as a cornerstone in the field of geometric inequalities and is a topic of interest in advanced mathematical research.
Xem video này để hiểu rõ hơn về mối quan hệ giữa chu vi và diện tích trong toán học và các ứng dụng thực tế.
Quan hệ giữa Chu vi & Diện tích là gì?
READ MORE:
Xem video này để tìm hiểu về mối quan hệ giữa chu vi và diện tích của các hình học, cùng với ứng dụng của nó trong thực tế.
Mối quan hệ giữa chu vi và diện tích