Area and Perimeter of Triangle Formula: Your Complete Guide

Area of a Triangle

The area \(A\) of a triangle can be calculated using different formulas depending on the information available. Here are the most common methods:

• Using Base and Height:

  If the base \(b\) and height \(h\) of a triangle are known, the area is given by:

  \[
  A = \frac{1}{2} \times b \times h
  \]

• Using Heron's Formula:

  If the lengths of all three sides \(a\), \(b\), and \(c\) are known, the area can be calculated using Heron's formula. First, calculate the semi-perimeter \(s\):

  \[
  s = \frac{a + b + c}{2}
  \]

  Then the area is given by:

  \[
  A = \sqrt{s(s - a)(s - b)(s - c)}
  \]

• Using Trigonometry:

  If two sides and the included angle are known, the area can be calculated as follows:

  \[
  A = \frac{1}{2} \times a \times b \times \sin(C)
  \]

  where \(a\) and \(b\) are the sides, and \(C\) is the included angle.

Perimeter of a Triangle

The perimeter \(P\) of a triangle is the sum of the lengths of its sides. For a triangle with sides \(a\), \(b\), and \(c\), the perimeter is given by:

\[
P = a + b + c
\]

Examples

• Example 1:

  Given a triangle with base \(b = 8\) units and height \(h = 5\) units, the area is:

  \[
  A = \frac{1}{2} \times 8 \times 5 = 20 \text{ square units}
  \]

• Example 2:

  Given a triangle with sides \(a = 3\) units, \(b = 4\) units, and \(c = 5\) units, first find the semi-perimeter:

  \[
  s = \frac{3 + 4 + 5}{2} = 6
  \]

  Then the area is:

  \[
  A = \sqrt{6(6 - 3)(6 - 4)(6 - 5)} = \sqrt{6 \times 3 \times 2 \times 1} = \sqrt{36} = 6 \text{ square units}
  \]

• Example 3:

  Given a triangle with sides \(a = 7\) units, \(b = 10\) units, and an included angle \(C = 45^\circ\), the area is:

  \[
  A = \frac{1}{2} \times 7 \times 10 \times \sin(45^\circ) = \frac{1}{2} \times 7 \times 10 \times \frac{\sqrt{2}}{2} = 24.75 \text{ square units}
  \]

Summary

• To calculate the area of a triangle, use the appropriate formula based on the given information (base and height, Heron's formula, or trigonometry).
• The perimeter of a triangle is simply the sum of the lengths of its sides.

The area and perimeter of a triangle are fundamental concepts in geometry, essential for various applications in mathematics, engineering, architecture, and more. Understanding these concepts allows for the accurate calculation of space and boundary measurements of triangular shapes. This guide will take you through the different formulas and methods to calculate the area and perimeter of a triangle, making it easy to solve problems involving triangles.

Triangles, being the simplest polygons, have unique properties that make their study both interesting and crucial. There are various types of triangles—equilateral, isosceles, and scalene—each with its characteristics. The methods for calculating the area and perimeter can differ based on the type of triangle and the information available.

In this section, we will cover:

• Basic Definitions: Understand the fundamental terms such as base, height, and sides of a triangle.
• Formulas for Area: Learn the different formulas to calculate the area using base and height, Heron's formula, and trigonometric principles.
• Formulas for Perimeter: Explore the simple method to determine the perimeter by summing the lengths of the sides.
• Examples and Applications: Step-by-step examples to illustrate the use of these formulas in solving real-world problems.

By mastering these formulas and concepts, you will be well-equipped to tackle a wide range of problems involving the measurement and analysis of triangles.

A triangle is a polygon with three edges and three vertices. It is one of the most fundamental shapes in geometry. The basic elements of a triangle include its sides, vertices, angles, base, and height. Understanding these elements is essential for calculating the area and perimeter of a triangle.

• Sides: A triangle has three sides, typically denoted as \(a\), \(b\), and \(c\). These sides can be of different lengths, leading to different types of triangles.
• Vertices: The points where the sides meet are called vertices. A triangle has three vertices, usually labeled as \(A\), \(B\), and \(C\).
• Angles: A triangle has three interior angles. The sum of these angles is always \(180^\circ\). These angles are typically denoted as \(\alpha\), \(\beta\), and \(\gamma\).
• Base and Height: The base of a triangle can be any one of its sides, often denoted as \(b\). The height (or altitude) is the perpendicular distance from the base to the opposite vertex, denoted as \(h\).

Types of Triangles

Triangles can be classified based on the lengths of their sides or the measures of their angles.

• By Sides:
  • Equilateral Triangle: All three sides are equal (\(a = b = c\)), and all three angles are \(60^\circ\).
  • Isosceles Triangle: Two sides are equal (\(a = b\)), and the angles opposite these sides are equal.
  • Scalene Triangle: All three sides and all three angles are different.
• By Angles:
  • Acute Triangle: All three angles are less than \(90^\circ\).
  • Right Triangle: One angle is exactly \(90^\circ\).
  • Obtuse Triangle: One angle is greater than \(90^\circ\).

Properties of Triangles

Some fundamental properties of triangles include:

• The sum of the interior angles of a triangle is always \(180^\circ\).
• The exterior angle of a triangle is equal to the sum of the two opposite interior angles.
• The length of any side of a triangle is less than the sum of the lengths of the other two sides (Triangle Inequality Theorem).

With these basic concepts in mind, we can move forward to explore the formulas for calculating the area and perimeter of a triangle.

The area of a triangle can be calculated using several methods, depending on the information available. Below are the most commonly used formulas:

Using Base and Height

When the base and height of a triangle are known, the area can be calculated using the formula:

\[
A = \frac{1}{2} \times b \times h
\]

where \(A\) is the area, \(b\) is the base, and \(h\) is the height of the triangle.

Heron's Formula

When the lengths of all three sides are known, the area can be found using Heron's formula. First, calculate the semi-perimeter (\(s\)) of the triangle:

\[
s = \frac{a + b + c}{2}
\]

where \(a\), \(b\), and \(c\) are the lengths of the sides. Then, the area is given by:

\[
A = \sqrt{s(s - a)(s - b)(s - c)}
\]

This formula is particularly useful for scalene triangles where the sides are of different lengths.

Using Trigonometry

When two sides and the included angle are known, the area can be calculated using the formula:

\[
A = \frac{1}{2} \times a \times b \times \sin(C)
\]

where \(a\) and \(b\) are the lengths of the sides, and \(C\) is the measure of the included angle.

Using Coordinates

If the vertices of the triangle are given in a coordinate plane as \((x_1, y_1)\), \((x_2, y_2)\), and \((x_3, y_3)\), the area can be calculated using the formula:

\[
A = \frac{1}{2} \left| x_1(y_2 - y_3) + x_2(y_3 - y_1) + x_3(y_1 - y_2) \right|
\]

Examples

Let's consider a few examples to illustrate these formulas:

• Example 1: Given a triangle with a base of 8 units and a height of 5 units, the area is:

  \[
  A = \frac{1}{2} \times 8 \times 5 = 20 \text{ square units}
  \]

• Example 2: Given a triangle with sides \(a = 3\), \(b = 4\), and \(c = 5\):

  First, find the semi-perimeter:

  \[
  s = \frac{3 + 4 + 5}{2} = 6
  \]

  Then, calculate the area using Heron's formula:

  \[
  A = \sqrt{6(6 - 3)(6 - 4)(6 - 5)} = \sqrt{6 \times 3 \times 2 \times 1} = \sqrt{36} = 6 \text{ square units}
  \]

• Example 3: Given a triangle with sides \(a = 7\) and \(b = 10\) with an included angle of \(45^\circ\):

  \[
  A = \frac{1}{2} \times 7 \times 10 \times \sin(45^\circ) = \frac{1}{2} \times 7 \times 10 \times \frac{\sqrt{2}}{2} = 24.75 \text{ square units}
  \]

These formulas and examples should provide a solid foundation for calculating the area of any triangle.

The most straightforward method to calculate the area of a triangle is by using its base and height. This method is particularly useful for right-angled and isosceles triangles, but it can be applied to any triangle where the base and height are known.

Step-by-Step Process

1. Identify the Base and Height:

   Choose one side of the triangle to be the base. The height is the perpendicular distance from the chosen base to the opposite vertex. In a right-angled triangle, one of the legs can be the base, and the other leg is the height.

2. Apply the Formula:

   Use the following formula to calculate the area:

   \[
   A = \frac{1}{2} \times b \times h
   \]

   where \(A\) is the area, \(b\) is the length of the base, and \(h\) is the height.

Example

Let's consider an example to illustrate this method:

• Given: A triangle with a base (\(b\)) of 10 units and a height (\(h\)) of 6 units.
• Step 1: Identify the base and height.

  In this case, the base is 10 units, and the height is 6 units.

• Step 2: Apply the formula.

  \[
  A = \frac{1}{2} \times 10 \times 6 = \frac{1}{2} \times 60 = 30 \text{ square units}
  \]

Special Cases

There are special cases where the base and height method can be used:

• Right-Angled Triangle:

  For a right-angled triangle, one leg can be taken as the base and the other as the height.

• Isosceles Triangle:

  For an isosceles triangle, if the base and height are known, the same formula applies.

• Equilateral Triangle:

  For an equilateral triangle, the height can be found using the Pythagorean theorem if the side length is known. The formula for the height (\(h\)) of an equilateral triangle with side length \(a\) is:

  \[
  h = \frac{\sqrt{3}}{2} \times a
  \]

  Then the area can be calculated as:

  \[
  A = \frac{1}{2} \times a \times h = \frac{1}{2} \times a \times \frac{\sqrt{3}}{2} \times a = \frac{\sqrt{3}}{4} \times a^2
  \]

Using the base and height to calculate the area of a triangle is a fundamental and widely applicable method in geometry.

Heron's formula is a useful method for calculating the area of a triangle when the lengths of all three sides are known. This formula is particularly helpful for scalene triangles where no height is given.

Step-by-Step Process

1. Calculate the Semi-Perimeter:

   First, find the semi-perimeter (\(s\)) of the triangle. The semi-perimeter is half of the perimeter of the triangle. If the sides of the triangle are \(a\), \(b\), and \(c\), the semi-perimeter is calculated as:

   \[
   s = \frac{a + b + c}{2}
   \]

2. Apply Heron's Formula:

   Once you have the semi-perimeter, use Heron's formula to find the area (\(A\)) of the triangle:

   \[
   A = \sqrt{s(s - a)(s - b)(s - c)}
   \]

   Here, \(s\) is the semi-perimeter, and \(a\), \(b\), and \(c\) are the lengths of the sides of the triangle.

Example

Let's consider an example to illustrate Heron's formula:

• Given: A triangle with sides \(a = 7\) units, \(b = 8\) units, and \(c = 9\) units.
• Step 1: Calculate the semi-perimeter.

  \[
  s = \frac{7 + 8 + 9}{2} = 12 \text{ units}
  \]

• Step 2: Apply Heron's formula.

  \[
  A = \sqrt{12(12 - 7)(12 - 8)(12 - 9)} = \sqrt{12 \times 5 \times 4 \times 3} = \sqrt{720} = 26.83 \text{ square units}
  \]

Special Cases

Heron's formula can be applied to any type of triangle, including equilateral, isosceles, and scalene triangles. Here are some special cases:

• Equilateral Triangle:

  For an equilateral triangle with all sides equal to \(a\), the semi-perimeter is:

  \[
  s = \frac{3a}{2}
  \]

  The area is then:

  \[
  A = \sqrt{\frac{3a}{2}\left(\frac{3a}{2} - a\right)\left(\frac{3a}{2} - a\right)\left(\frac{3a}{2} - a\right)} = \sqrt{\frac{3a}{2} \times \frac{a}{2} \times \frac{a}{2} \times \frac{a}{2}} = \sqrt{\frac{3a^4}{16}} = \frac{\sqrt{3}a^2}{4}
  \]

• Isosceles Triangle:

  For an isosceles triangle with two sides equal to \(a\) and the base \(b\), the semi-perimeter is:

  \[
  s = \frac{2a + b}{2}
  \]

  The area is then:

  \[
  A = \sqrt{\frac{2a + b}{2}\left(\frac{2a + b}{2} - a\right)\left(\frac{2a + b}{2} - a\right)\left(\frac{2a + b}{2} - b\right)}
  \]

Heron's formula is a versatile and powerful tool for finding the area of a triangle when the side lengths are known, making it an essential part of geometry.

Trigonometry offers several powerful methods for calculating the area of a triangle, especially when the lengths of two sides and the included angle are known. These methods are particularly useful in non-right-angled triangles.

Step-by-Step Process

1. Identify the Known Values:

   Determine which sides and angles are known. For instance, if two sides \(a\) and \(b\) and the included angle \(C\) are known, you can use the trigonometric area formula.

2. Apply the Trigonometric Area Formula:

   Use the formula:

   \[
   A = \frac{1}{2} \times a \times b \times \sin(C)
   \]

   where \(A\) is the area, \(a\) and \(b\) are the lengths of the sides, and \(C\) is the included angle between these sides.

Example

Let's consider an example to illustrate this method:

• Given: A triangle with sides \(a = 7\) units and \(b = 10\) units, and the included angle \(C = 45^\circ\).
• Step 1: Identify the known values.

  In this case, \(a = 7\) units, \(b = 10\) units, and \(C = 45^\circ\).

• Step 2: Apply the trigonometric area formula.

  \[
  A = \frac{1}{2} \times 7 \times 10 \times \sin(45^\circ) = \frac{1}{2} \times 7 \times 10 \times \frac{\sqrt{2}}{2} = 24.75 \text{ square units}
  \]

Special Cases

There are special cases where trigonometric formulas can be applied effectively:

• When All Angles Are Known:

  If all angles and one side are known, you can use the Law of Sines to find the missing sides and then apply the trigonometric area formula.

• Right-Angled Triangles:

  For right-angled triangles, the area can also be found using trigonometric functions of the known angles.

Using the Law of Sines

The Law of Sines can help find the area if one side and the corresponding angle are known, along with another angle. The area (\(A\)) can be found using:

\[
A = \frac{a^2 \cdot \sin(B) \cdot \sin(C)}{2 \cdot \sin(A)}
\]

where \(a\) is the known side, and \(A\), \(B\), and \(C\) are the angles.

Examples

Let's consider another example using the Law of Sines:

• Given: A triangle with side \(a = 8\) units, angle \(A = 30^\circ\), and angle \(B = 60^\circ\).
• Step 1: Calculate the remaining angle \(C\).

  \[
  C = 180^\circ - A - B = 180^\circ - 30^\circ - 60^\circ = 90^\circ
  \]

• Step 2: Apply the Law of Sines to find another side if needed. For area calculation directly:

  \[
  A = \frac{8^2 \cdot \sin(60^\circ) \cdot \sin(90^\circ)}{2 \cdot \sin(30^\circ)} = \frac{64 \cdot \frac{\sqrt{3}}{2} \cdot 1}{2 \cdot \frac{1}{2}} = 64 \cdot \frac{\sqrt{3}}{2} = 32\sqrt{3} \text{ square units}
  \]

Using trigonometry to calculate the area of a triangle is a versatile method that can handle various triangle configurations, making it a powerful tool in geometry.

The perimeter of a triangle is the total length of its sides. There are different ways to calculate the perimeter depending on the type of triangle and the information available. Here, we will discuss the general formula and specific formulas for different types of triangles.

General Formula

The most straightforward way to calculate the perimeter of a triangle is to sum the lengths of its three sides. If the sides of a triangle are denoted as \(a\), \(b\), and \(c\), then the formula for the perimeter \(P\) is:

\[ P = a + b + c \]

Perimeter of Special Types of Triangles

Equilateral Triangle

An equilateral triangle has all three sides equal. If each side is of length \(a\), the formula for the perimeter \(P\) is:

\[ P = 3a \]

Isosceles Triangle

An isosceles triangle has two sides of equal length. If the equal sides are of length \(a\) and the base is of length \(b\), the formula for the perimeter \(P\) is:

\[ P = 2a + b \]

Scalene Triangle

A scalene triangle has all sides of different lengths. As with the general formula, if the sides are \(a\), \(b\), and \(c\), the perimeter \(P\) is:

\[ P = a + b + c \]

Examples

Let's look at a few examples to understand how these formulas are applied:

• Example 1: An equilateral triangle with side length \(5\) units. The perimeter is: \[ P = 3 \times 5 = 15 \, \text{units} \]
• Example 2: An isosceles triangle with equal sides of length \(7\) units and a base of \(10\) units. The perimeter is: \[ P = 2 \times 7 + 10 = 24 \, \text{units} \]
• Example 3: A scalene triangle with sides of length \(3\), \(4\), and \(5\) units. The perimeter is: \[ P = 3 + 4 + 5 = 12 \, \text{units} \]

Practice Problems

Try solving these practice problems to test your understanding:

1. Find the perimeter of an equilateral triangle with each side measuring \(8\) units.
2. An isosceles triangle has two sides of \(6\) units each and a base of \(8\) units. What is its perimeter?
3. Calculate the perimeter of a scalene triangle with sides \(9\) units, \(12\) units, and \(15\) units.

Triangles come in various forms, each with unique properties and specific formulas for calculating their area and perimeter. Here, we discuss three special types of triangles: equilateral, isosceles, and scalene.

Equilateral Triangle

An equilateral triangle has all three sides of equal length and all three angles equal to \(60^\circ\).

• Perimeter: If the side length is \(a\), the perimeter \(P\) is: \[ P = 3a \]
• Area: The area \(A\) can be calculated using the formula: \[ A = \frac{\sqrt{3}}{4} a^2 \]

Isosceles Triangle

An isosceles triangle has two sides of equal length and two equal angles opposite these sides.

• Perimeter: If the equal sides are \(a\) and the base is \(b\), the perimeter \(P\) is: \[ P = 2a + b \]
• Area: The area \(A\) can be calculated using the formula: \[ A = \frac{b \sqrt{4a^2 - b^2}}{4} \]

Scalene Triangle

A scalene triangle has all sides of different lengths and all angles of different measures.

• Perimeter: If the sides are \(a\), \(b\), and \(c\), the perimeter \(P\) is: \[ P = a + b + c \]
• Area: The area \(A\) can be calculated using Heron's formula: \[ s = \frac{a + b + c}{2} \] \[ A = \sqrt{s(s-a)(s-b)(s-c)} \]

Examples

Let's look at some examples to illustrate the calculations:

• Example 1: An equilateral triangle with side length \(6\) units.
  • Perimeter: \[ P = 3 \times 6 = 18 \, \text{units} \]
  • Area: \[ A = \frac{\sqrt{3}}{4} \times 6^2 = 9\sqrt{3} \, \text{square units} \]
• Example 2: An isosceles triangle with equal sides of \(5\) units and a base of \(8\) units.
  • Perimeter: \[ P = 2 \times 5 + 8 = 18 \, \text{units} \]
  • Area: \[ A = \frac{8 \sqrt{4 \times 5^2 - 8^2}}{4} = 12 \, \text{square units} \]
• Example 3: A scalene triangle with sides \(7\) units, \(8\) units, and \(5\) units.
  • Perimeter: \[ P = 7 + 8 + 5 = 20 \, \text{units} \]
  • Area: \[ s = \frac{7 + 8 + 5}{2} = 10 \] \[ A = \sqrt{10(10-7)(10-8)(10-5)} = \sqrt{10 \times 3 \times 2 \times 5} = \sqrt{300} = 10\sqrt{3} \, \text{square units} \]

Practice Problems

Try solving these practice problems to reinforce your understanding:

1. Find the perimeter and area of an equilateral triangle with side length \(10\) units.
2. An isosceles triangle has sides \(6\) units, \(6\) units, and \(8\) units. Calculate its perimeter and area.
3. Determine the perimeter and area of a scalene triangle with sides \(9\) units, \(12\) units, and \(15\) units.

An equilateral triangle is a special type of triangle where all three sides are of equal length and all three internal angles are equal to \(60^\circ\). This symmetry provides unique and straightforward formulas for calculating both the area and perimeter.

Properties of an Equilateral Triangle

• All sides are of equal length \(a\).
• All internal angles are equal to \(60^\circ\).
• It is both a regular polygon and a convex polygon.

Perimeter

The perimeter of an equilateral triangle is simply three times the length of one side. If the length of each side is \(a\), the perimeter \(P\) is given by:

\[ P = 3a \]

Area

The area of an equilateral triangle can be calculated using the formula:

\[ A = \frac{\sqrt{3}}{4} a^2 \]

Here’s how you derive this formula:

1. Consider an equilateral triangle with side length \(a\).
2. Draw a perpendicular height from one vertex to the midpoint of the opposite side, bisecting the equilateral triangle into two 30-60-90 right triangles.
3. In a 30-60-90 triangle, the ratio of the sides is \(1 : \sqrt{3} : 2\). Therefore, the height \(h\) of the equilateral triangle can be expressed as: \[ h = \frac{\sqrt{3}}{2} a \]
4. The area \(A\) of the triangle is then: \[ A = \frac{1}{2} \times \text{base} \times \text{height} = \frac{1}{2} \times a \times \frac{\sqrt{3}}{2} a = \frac{\sqrt{3}}{4} a^2 \]

Examples

Let’s go through a few examples to solidify the concepts:

• Example 1: An equilateral triangle with side length \(4\) units.
  • Perimeter: \[ P = 3 \times 4 = 12 \, \text{units} \]
  • Area: \[ A = \frac{\sqrt{3}}{4} \times 4^2 = 4\sqrt{3} \, \text{square units} \]
• Example 2: An equilateral triangle with side length \(10\) units.
  • Perimeter: \[ P = 3 \times 10 = 30 \, \text{units} \]
  • Area: \[ A = \frac{\sqrt{3}}{4} \times 10^2 = 25\sqrt{3} \, \text{square units} \]

Practice Problems

Try solving these practice problems to enhance your understanding:

1. Calculate the perimeter and area of an equilateral triangle with a side length of \(7\) units.
2. Find the perimeter and area of an equilateral triangle with a side length of \(12\) units.
3. An equilateral triangle has a perimeter of \(45\) units. Determine the side length and the area of the triangle.

An isosceles triangle is a type of triangle that has two sides of equal length. These two sides are called the legs, and the third side is called the base. The angles opposite the equal sides are also equal.

Properties of an Isosceles Triangle

• Two sides of equal length (\(a\)).
• The base (\(b\)) is different in length.
• Two equal angles opposite the equal sides.

Perimeter

The perimeter of an isosceles triangle is the sum of the lengths of all its sides. If the lengths of the equal sides are \(a\) and the base is \(b\), the perimeter \(P\) is given by:

\[ P = 2a + b \]

Area

The area of an isosceles triangle can be calculated using the base and height. The height (\(h\)) can be found using the Pythagorean theorem if it's not directly given. The formula for the area \(A\) is:

\[ A = \frac{1}{2} \times b \times h \]

To find the height (\(h\)) in terms of \(a\) and \(b\):

1. Draw a perpendicular from the vertex opposite the base to the midpoint of the base, splitting the isosceles triangle into two right triangles.
2. The length of the base is divided into two equal parts, each \(\frac{b}{2}\).
3. Using the Pythagorean theorem in one of the right triangles: \[ h = \sqrt{a^2 - \left(\frac{b}{2}\right)^2} \]

Substituting \(h\) into the area formula gives:
\[ A = \frac{1}{2} \times b \times \sqrt{a^2 - \left(\frac{b}{2}\right)^2} \]

Examples

Let’s go through some examples to illustrate the calculations:

• Example 1: An isosceles triangle with equal sides \(5\) units and a base \(8\) units.
  • Perimeter: \[ P = 2 \times 5 + 8 = 18 \, \text{units} \]
  • Area: \[ h = \sqrt{5^2 - \left(\frac{8}{2}\right)^2} = \sqrt{25 - 16} = \sqrt{9} = 3 \] \[ A = \frac{1}{2} \times 8 \times 3 = 12 \, \text{square units} \]
• Example 2: An isosceles triangle with equal sides \(10\) units and a base \(12\) units.
  • Perimeter: \[ P = 2 \times 10 + 12 = 32 \, \text{units} \]
  • Area: \[ h = \sqrt{10^2 - \left(\frac{12}{2}\right)^2} = \sqrt{100 - 36} = \sqrt{64} = 8 \] \[ A = \frac{1}{2} \times 12 \times 8 = 48 \, \text{square units} \]

Practice Problems

Try solving these practice problems to enhance your understanding:

1. Calculate the perimeter and area of an isosceles triangle with equal sides \(7\) units and base \(10\) units.
2. An isosceles triangle has equal sides of \(9\) units and a base of \(12\) units. Find its perimeter and area.
3. Determine the perimeter and area of an isosceles triangle with equal sides \(6\) units and a base of \(8\) units.

A scalene triangle is a type of triangle where all three sides have different lengths and all three internal angles are different. This uniqueness requires a general approach for calculating the area and perimeter.

Properties of a Scalene Triangle

• All sides have different lengths (\(a\), \(b\), and \(c\)).
• All internal angles are different.
• No sides or angles are equal.

Perimeter

The perimeter of a scalene triangle is the sum of the lengths of all its sides. If the sides are \(a\), \(b\), and \(c\), the perimeter \(P\) is given by:

\[ P = a + b + c \]

Area

The area of a scalene triangle can be calculated using Heron's formula, which involves the semi-perimeter (\(s\)). The steps are as follows:

1. Calculate the semi-perimeter: \[ s = \frac{a + b + c}{2} \]
2. Use Heron's formula to find the area \(A\): \[ A = \sqrt{s(s-a)(s-b)(s-c)} \]

Examples

Let’s go through some examples to illustrate the calculations:

• Example 1: A scalene triangle with sides \(7\) units, \(8\) units, and \(5\) units.
  • Perimeter: \[ P = 7 + 8 + 5 = 20 \, \text{units} \]
  • Area: \[ s = \frac{7 + 8 + 5}{2} = 10 \] \[ A = \sqrt{10(10-7)(10-8)(10-5)} = \sqrt{10 \times 3 \times 2 \times 5} = \sqrt{300} = 10\sqrt{3} \, \text{square units} \]
• Example 2: A scalene triangle with sides \(9\) units, \(12\) units, and \(15\) units.
  • Perimeter: \[ P = 9 + 12 + 15 = 36 \, \text{units} \]
  • Area: \[ s = \frac{9 + 12 + 15}{2} = 18 \] \[ A = \sqrt{18(18-9)(18-12)(18-15)} = \sqrt{18 \times 9 \times 6 \times 3} = \sqrt{2916} = 54 \, \text{square units} \]

Practice Problems

Try solving these practice problems to enhance your understanding:

1. Calculate the perimeter and area of a scalene triangle with sides \(11\) units, \(14\) units, and \(15\) units.
2. A scalene triangle has sides \(6\) units, \(8\) units, and \(10\) units. Find its perimeter and area.
3. Determine the perimeter and area of a scalene triangle with sides \(13\) units, \(14\) units, and \(15\) units.

Understanding the formulas for calculating the area and perimeter of different types of triangles is essential. Below are detailed examples and practice problems to help reinforce these concepts.

Examples

Let's explore some examples for equilateral, isosceles, and scalene triangles.

Equilateral Triangle

Example: An equilateral triangle with a side length of \(6\) units.

• Perimeter: \[ P = 3a = 3 \times 6 = 18 \, \text{units} \]
• Area: \[ A = \frac{\sqrt{3}}{4} a^2 = \frac{\sqrt{3}}{4} \times 6^2 = \frac{\sqrt{3}}{4} \times 36 = 9\sqrt{3} \, \text{square units} \]

Isosceles Triangle

Example: An isosceles triangle with equal sides of \(5\) units and a base of \(8\) units.

• Perimeter: \[ P = 2a + b = 2 \times 5 + 8 = 18 \, \text{units} \]
• Area: \[ h = \sqrt{a^2 - \left(\frac{b}{2}\right)^2} = \sqrt{5^2 - \left(\frac{8}{2}\right)^2} = \sqrt{25 - 16} = \sqrt{9} = 3 \, \text{units} \] \[ A = \frac{1}{2} \times b \times h = \frac{1}{2} \times 8 \times 3 = 12 \, \text{square units} \]

Scalene Triangle

Example: A scalene triangle with sides \(7\) units, \(8\) units, and \(5\) units.

• Perimeter: \[ P = a + b + c = 7 + 8 + 5 = 20 \, \text{units} \]
• Area: \[ s = \frac{a + b + c}{2} = \frac{7 + 8 + 5}{2} = 10 \] \[ A = \sqrt{s(s-a)(s-b)(s-c)} = \sqrt{10(10-7)(10-8)(10-5)} = \sqrt{10 \times 3 \times 2 \times 5} = \sqrt{300} = 10\sqrt{3} \, \text{square units} \]

Practice Problems

Try solving these practice problems to enhance your understanding:

1. Find the perimeter and area of an equilateral triangle with a side length of \(10\) units.
2. An isosceles triangle has sides of \(7\) units, \(7\) units, and \(10\) units. Calculate its perimeter and area.
3. Determine the perimeter and area of a scalene triangle with sides \(9\) units, \(12\) units, and \(15\) units.
4. Calculate the area of an isosceles triangle with equal sides \(13\) units and a base \(10\) units.
5. Find the area of a scalene triangle with sides \(8\) units, \(15\) units, and \(17\) units.

By working through these examples and problems, you'll gain a deeper understanding of how to calculate the area and perimeter for various types of triangles.

Below are some detailed example calculations for finding the area and perimeter of different types of triangles. These examples will help illustrate how to apply the formulas in practice.

Equilateral Triangle

Example: Calculate the perimeter and area of an equilateral triangle with a side length of \(a = 6\) units.

• Perimeter: \[ P = 3a = 3 \times 6 = 18 \, \text{units} \]
• Area: \[ A = \frac{\sqrt{3}}{4} a^2 = \frac{\sqrt{3}}{4} \times 6^2 = \frac{\sqrt{3}}{4} \times 36 = 9\sqrt{3} \, \text{square units} \]

Isosceles Triangle

Example: Calculate the perimeter and area of an isosceles triangle with equal sides \(a = 5\) units and a base \(b = 8\) units.

• Perimeter: \[ P = 2a + b = 2 \times 5 + 8 = 18 \, \text{units} \]
• Area: \[ h = \sqrt{a^2 - \left(\frac{b}{2}\right)^2} = \sqrt{5^2 - \left(\frac{8}{2}\right)^2} = \sqrt{25 - 16} = \sqrt{9} = 3 \, \text{units} \] \[ A = \frac{1}{2} \times b \times h = \frac{1}{2} \times 8 \times 3 = 12 \, \text{square units} \]

Scalene Triangle

Example: Calculate the perimeter and area of a scalene triangle with sides \(a = 7\) units, \(b = 8\) units, and \(c = 5\) units.

• Perimeter: \[ P = a + b + c = 7 + 8 + 5 = 20 \, \text{units} \]
• Area: \[ s = \frac{a + b + c}{2} = \frac{7 + 8 + 5}{2} = 10 \] \[ A = \sqrt{s(s-a)(s-b)(s-c)} = \sqrt{10(10-7)(10-8)(10-5)} = \sqrt{10 \times 3 \times 2 \times 5} = \sqrt{300} = 10\sqrt{3} \, \text{square units} \]

Right Triangle

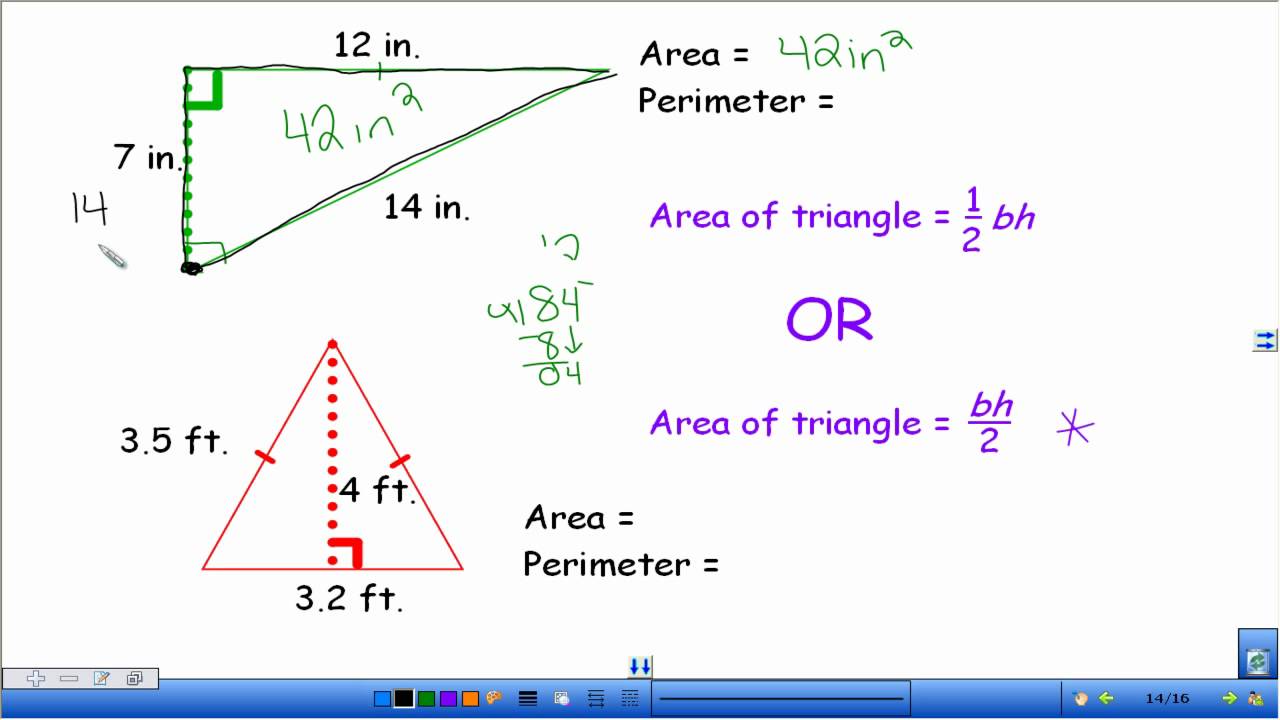
Example: Calculate the perimeter and area of a right triangle with legs \(a = 3\) units and \(b = 4\) units.

• Perimeter: \[ c = \sqrt{a^2 + b^2} = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5 \, \text{units} \] \[ P = a + b + c = 3 + 4 + 5 = 12 \, \text{units} \]
• Area: \[ A = \frac{1}{2} \times a \times b = \frac{1}{2} \times 3 \times 4 = \frac{1}{2} \times 12 = 6 \, \text{square units} \]

Practice Problems

Try solving these practice problems to enhance your understanding:

1. Find the perimeter and area of an equilateral triangle with a side length of \(10\) units.
2. An isosceles triangle has sides of \(7\) units, \(7\) units, and \(10\) units. Calculate its perimeter and area.
3. Determine the perimeter and area of a scalene triangle with sides \(9\) units, \(12\) units, and \(15\) units.
4. Calculate the area of an isosceles triangle with equal sides \(13\) units and a base \(10\) units.
5. Find the area of a scalene triangle with sides \(8\) units, \(15\) units, and \(17\) units.
6. Find the perimeter and area of a right triangle with legs \(6\) units and \(8\) units.

By working through these examples and problems, you'll gain a deeper understanding of how to calculate the area and perimeter for various types of triangles.

Below are some practice problems to help reinforce your understanding of calculating the area and perimeter of different types of triangles. Work through each problem step by step and use the provided formulas.

Equilateral Triangle

1. Calculate the perimeter and area of an equilateral triangle with a side length of \(12\) units.
2. An equilateral triangle has a side length of \(7\) units. Find its perimeter and area.

Isosceles Triangle

1. Find the perimeter and area of an isosceles triangle with equal sides of \(9\) units and a base of \(12\) units.
2. Calculate the perimeter and area of an isosceles triangle with equal sides of \(8\) units and a base of \(6\) units.

Scalene Triangle

1. Determine the perimeter and area of a scalene triangle with sides \(11\) units, \(13\) units, and \(15\) units.
2. A scalene triangle has sides \(10\) units, \(14\) units, and \(18\) units. Calculate its perimeter and area.

Right Triangle

1. Find the perimeter and area of a right triangle with legs \(6\) units and \(8\) units.
2. Calculate the perimeter and area of a right triangle with legs \(5\) units and \(12\) units.

Additional Problems

1. An equilateral triangle has a perimeter of \(24\) units. Calculate its side length and area.
2. An isosceles triangle has a perimeter of \(30\) units and each of its equal sides is \(11\) units. Find the base length and the area.
3. A scalene triangle has a perimeter of \(40\) units. If two of its sides are \(12\) units and \(15\) units, find the length of the third side and the area.
4. Calculate the height of an isosceles triangle with equal sides of \(13\) units and a base of \(10\) units.
5. Determine the area of a scalene triangle with sides \(8\) units, \(15\) units, and \(17\) units using Heron's formula.

Working through these problems will give you a solid understanding of how to apply the formulas for the area and perimeter of triangles. Make sure to review your calculations and ensure accuracy at each step.

Understanding the area and perimeter of triangles is not just a mathematical exercise; these concepts have numerous practical applications in real life. Here are some examples:

Triangles in Architecture

Triangles are a fundamental shape in architecture due to their inherent stability and strength. They are used in the design of buildings, bridges, and other structures.

• Roof Trusses: Triangular trusses are used in roof construction to distribute weight evenly and provide strong support.
• Bridge Design: Triangular shapes are used in bridges to ensure even load distribution and to enhance structural integrity.
• Geodesic Domes: These domes are constructed using a network of triangles, providing a lightweight yet strong structure.

Triangles in Engineering

Engineering fields also make extensive use of triangles, especially in areas such as mechanical and civil engineering.

• Structural Analysis: Engineers use the properties of triangles to analyze forces and stresses in structures, ensuring safety and durability.
• Component Design: Triangular components are often used in machinery and vehicles to provide stability and strength.
• Surveying: Triangulation is a method used by engineers to determine distances and map out areas accurately.

Triangles in Nature

Triangles are not just man-made; they are also found abundantly in nature, where their geometric properties provide various advantages.

• Crystal Structures: Many minerals and crystals form naturally in triangular or tetrahedral shapes due to their molecular bonds.
• Biological Forms: The structure of many biological forms, such as honeycombs and certain leaves, incorporates triangles for optimal space utilization and strength.
• Natural Formations: Triangular shapes can be observed in mountains, rock formations, and other geological structures, contributing to their stability.

Triangles in Art and Design

Triangles are a popular element in art and design due to their visual appeal and versatility.

• Graphic Design: Triangles are used to create dynamic and interesting compositions in graphic design.
• Patterns and Textiles: Triangular patterns are commonly used in textiles, tiling, and other decorative arts.
• Visual Balance: Triangles are used in artworks to create a sense of balance and harmony.

Practical Everyday Uses

In everyday life, understanding triangles can help in various practical scenarios.

• Home Improvement: Calculating the area and perimeter of triangular spaces can assist in tasks such as tiling, flooring, and landscaping.
• Crafts and DIY Projects: Triangular shapes are often used in crafts, DIY projects, and furniture design for both aesthetic and structural purposes.
• Navigation: Triangulation is a method used in navigation and mapping to pinpoint locations accurately.

By recognizing and applying the properties of triangles in these real-life contexts, we can better appreciate the importance of these geometric principles and utilize them effectively in various fields.

Triangles play a crucial role in architecture due to their inherent stability and strength. They are fundamental in the design and construction of various structures, from bridges to buildings, owing to their ability to evenly distribute weight and withstand external forces.

One of the primary ways triangles are used in architecture is through trusses. Trusses are frameworks consisting of triangular units constructed with straight members. This design allows for the efficient distribution of loads, making them ideal for supporting roofs, bridges, and towers.

• Roof Trusses: In roofing, trusses provide a lightweight yet strong framework. They are designed to bear the load of the roof and withstand various environmental factors like wind and snow.
• Bridge Trusses: Triangular configurations are also prevalent in bridge construction. Designs like the Pratt and Warren trusses use triangles to distribute the weight of vehicles and pedestrians, ensuring structural integrity and longevity.

Additionally, the triangle's geometric properties make it a preferred shape in the design of various architectural elements:

• Domes and Arches: The geodesic dome, popularized by architect Buckminster Fuller, is composed of a network of triangles that create a lightweight, strong, and efficient structure.
• Space Frames: Space frames utilize a network of interconnected triangles to form a rigid, lightweight, and versatile framework, often used in large-span structures like sports arenas and exhibition halls.

The stability of triangles comes from their ability to maintain their shape under pressure, unlike other polygons that can be deformed without altering the length of their sides. This characteristic is known as rigidity, making triangles an essential component in creating stable and enduring architectural designs.

Mathematically, the area and perimeter of triangles are crucial for calculating the materials needed and ensuring precise construction:

• The area of a triangle is calculated using various formulas depending on the known dimensions. For instance, using the base and height: \( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \). For a triangle with all three sides known, Heron's formula is used: \( \text{Area} = \sqrt{s(s-a)(s-b)(s-c)} \), where \( s \) is the semi-perimeter.
• The perimeter is simply the sum of all its sides: \( \text{Perimeter} = a + b + c \).

Understanding these properties and formulas allows architects and engineers to design structures that are both aesthetically pleasing and structurally sound, demonstrating the indispensable role of triangles in architecture.

Triangles are one of the most fundamental shapes found in nature, providing structural integrity and aesthetic appeal across various natural formations. The inherent stability of triangles makes them prevalent in natural structures ranging from the microscopic level to large-scale geological formations.

Structural Stability

The triangular shape is incredibly stable and is often used by nature to provide structural support. This is because a triangle, unlike other polygons, is rigid and does not change shape unless its sides are altered. This property is leveraged by nature in several ways:

• Honeycombs: Bees construct hexagonal honeycombs, where each hexagon can be divided into six equilateral triangles. This division allows for efficient use of space and structural strength, optimizing storage for honey while using the least amount of wax.
• Crystals: Many minerals and crystals form triangular patterns at the microscopic level. For instance, quartz crystals often display triangular faces, reflecting the molecular arrangement that gives the crystal its strength and stability.

Geological Formations

Triangles are also visible in larger natural formations such as mountains and river deltas. These formations often exhibit triangular profiles due to erosion and sediment deposition processes.

• Mountains: The sides of mountains often form triangular shapes, with the base being the widest part and the peak representing the apex. This triangular shape provides stability and distributes the mountain's mass efficiently.
• River Deltas: Triangular shapes are also evident in river deltas where sediment is deposited as the river flows into the sea. The delta spreads out in a triangular pattern, optimizing the deposition area.

Biological Structures

Triangles can also be found in various biological structures, contributing to their strength and functionality:

• Leaves: Some leaves, such as those of the maple tree, have a triangular shape that allows for efficient sunlight capture and water runoff.
• Skeletons: The human pelvis and the bones of many animals form triangular structures, which provide both strength and flexibility necessary for movement and support.

Mathematical Applications

The geometric properties of triangles are utilized in nature for various functional purposes:

• Navigation: Animals, such as birds and fish, often move in triangular formations. This not only reduces drag but also improves energy efficiency during migration.
• Vision: The triangular arrangement of photoreceptor cells in the eyes of certain animals allows for more efficient light capture and better vision.

In summary, triangles are a crucial shape in nature, providing stability, strength, and efficiency across various natural and biological structures. Their unique geometric properties make them an essential component of both microscopic and macroscopic natural formations.

In this section, we delve into advanced concepts related to the area and perimeter of triangles, including their applications in coordinate geometry and connections to other geometric shapes.

Area of Triangles in Coordinate Geometry

To find the area of a triangle when the vertices are given in the coordinate plane, we use the following formula:

\[ A = \frac{1}{2} \left| x_1(y_2 - y_3) + x_2(y_3 - y_1) + x_3(y_1 - y_2) \right| \]

Where \((x_1, y_1)\), \((x_2, y_2)\), and \((x_3, y_3)\) are the coordinates of the triangle's vertices. This formula is derived from the determinant of a matrix and represents the absolute value of the sum of the areas of trapezoids formed by the triangle and the coordinate axes.

Perimeter of Triangles in Coordinate Geometry

The perimeter of a triangle in the coordinate plane is found by calculating the distances between each pair of vertices and summing them up:

\[ P = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} + \sqrt{(x_3 - x_2)^2 + (y_3 - y_2)^2} + \sqrt{(x_1 - x_3)^2 + (y_1 - y_3)^2} \]

Where \((x_1, y_1)\), \((x_2, y_2)\), and \((x_3, y_3)\) are the coordinates of the vertices. This method uses the distance formula to find the length of each side.

Area of a Triangle Using Vector Cross Product

In vector geometry, the area of a triangle formed by vectors \(\mathbf{u}\) and \(\mathbf{v}\) can be found using the cross product:

\[ A = \frac{1}{2} \|\mathbf{u} \times \mathbf{v}\| \]

Where \(\mathbf{u}\) and \(\mathbf{v}\) are vectors corresponding to two sides of the triangle, and \(\|\mathbf{u} \times \mathbf{v}\|\) is the magnitude of their cross product. This formula is particularly useful in physics and engineering.

Heron's Formula and Its Applications

Heron's formula allows the calculation of the area of a triangle when all three side lengths are known. Given the sides \(a\), \(b\), and \(c\), the area \(A\) is:

\[ A = \sqrt{s(s - a)(s - b)(s - c)} \]

Where \(s\) is the semi-perimeter:

\[ s = \frac{a + b + c}{2} \]

This formula is beneficial in various fields where only the side lengths are known, such as surveying and architecture.

Ellipses and Triangles with Fixed Perimeters

A triangle with a fixed perimeter can have varying areas. An interesting property is that when such a triangle is modified by moving one of its vertices while keeping the perimeter constant, the locus of the vertex forms an ellipse. This property has applications in mechanical engineering and design, particularly in mechanisms that involve linkage systems.

Conclusion

These advanced topics provide a deeper understanding of triangles and their properties in various mathematical and practical contexts. Mastery of these concepts can be particularly useful in fields such as physics, engineering, architecture, and computer graphics.