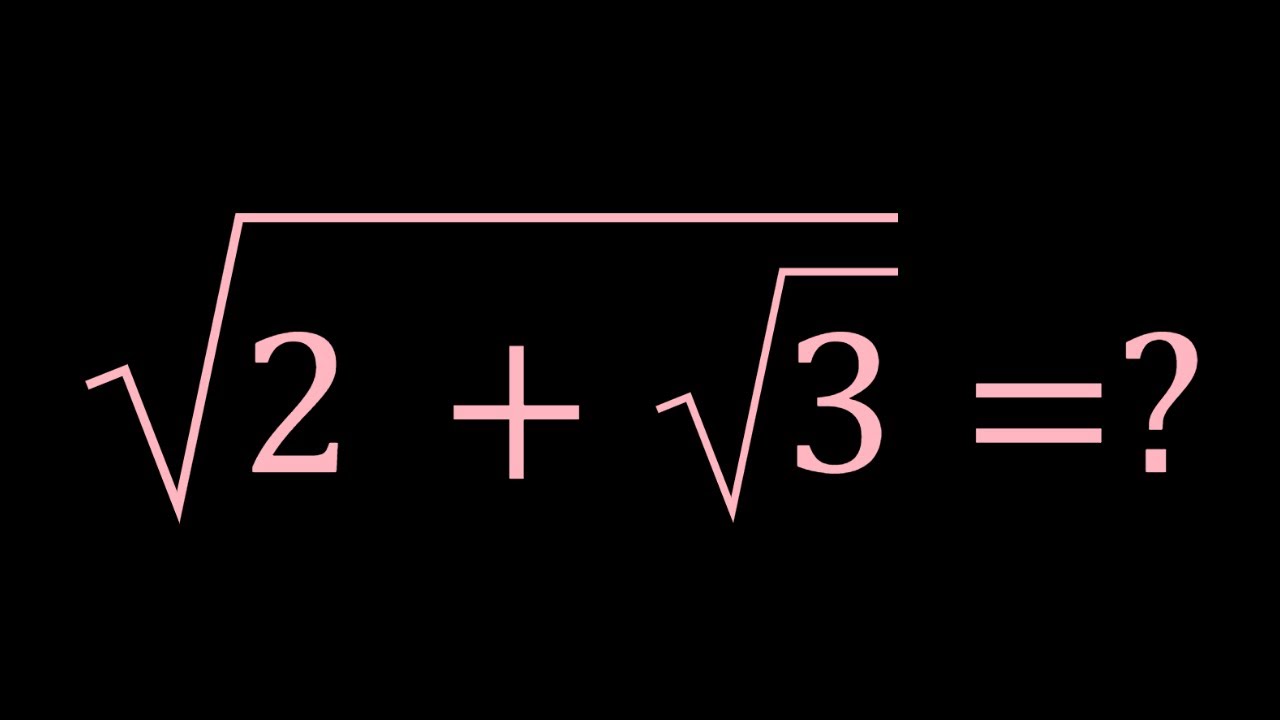Topic 2 square root of 10: Discover the fascinating world of the mathematical expression \(2 \sqrt{10}\). In this article, we delve into its calculation, properties, and practical applications across various fields. Learn how this intriguing number appears in geometry, physics, and engineering, and why understanding it can enhance your mathematical knowledge and problem-solving skills.
Table of Content
- Calculation of 2 Square Root of 10
- Introduction
- Definition of Square Roots
- Mathematical Representation of 2 Square Root of 10
- Decimal Approximation
- Properties of 2 Square Root of 10
- Applications in Geometry
- Applications in Physics
- Applications in Engineering
- Comparison with Other Square Roots
- Visual Representation
- Common Misconceptions
- FAQs
- YOUTUBE: Xem video hướng dẫn cách tính căn bậc hai của biểu thức (7 + 2√10) để giải quyết các bài toán liên quan đến từ khóa '2 căn bậc hai của 10'.
Calculation of 2 Square Root of 10
The expression \(2 \sqrt{10}\) represents two times the square root of ten. Below is a detailed breakdown of this mathematical expression.
Step-by-Step Calculation
- Identify the square root of 10.
\(\sqrt{10}\) is an irrational number, approximately equal to 3.16227766016838.
- Multiply this value by 2.
\(2 \times \sqrt{10} = 2 \times 3.16227766016838 \approx 6.32455532033676\)
Result
Therefore, the value of \(2 \sqrt{10}\) is approximately 6.32455532033676.
Properties of the Expression
- Irrational Number: The result is an irrational number because it involves the square root of a non-perfect square.
- Decimal Approximation: The decimal representation of \(2 \sqrt{10}\) goes on infinitely without repeating.
- Real Number: Both 2 and \(\sqrt{10}\) are real numbers, making their product a real number.
Applications
This value can appear in various contexts, such as geometry, physics, and engineering, where square roots and their multiples are often used.
Conclusion
The expression \(2 \sqrt{10}\) provides a useful calculation in mathematics and applied sciences, demonstrating the relationship between multiplication and square roots.
READ MORE:
Introduction
The expression \(2 \sqrt{10}\) represents a mathematical calculation that combines multiplication with the square root operation. This number has significance in various mathematical contexts and applications. In this section, we will explore the basic concepts and importance of \(2 \sqrt{10}\) in detail.
First, let us break down the components of the expression:
- \(\sqrt{10}\): The square root of 10, which is an irrational number.
- 2: A multiplier that scales the value of \(\sqrt{10}\).
When combined, these elements form \(2 \sqrt{10}\), which can be calculated as follows:
- Calculate the square root of 10.
\(\sqrt{10} \approx 3.16227766016838\)
- Multiply the result by 2.
\(2 \times 3.16227766016838 \approx 6.32455532033676\)
The value of \(2 \sqrt{10}\) is thus approximately 6.32455532033676.
This expression is not only a mathematical curiosity but also has practical applications in various fields such as geometry, physics, and engineering. Understanding \(2 \sqrt{10}\) helps in solving complex problems and enhances overall mathematical comprehension.
Definition of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is one of the fundamental operations in mathematics and is denoted by the radical symbol \(\sqrt{}\). For any positive number \(x\), the square root of \(x\) is written as \(\sqrt{x}\), and satisfies the equation:
\[
\sqrt{x} \times \sqrt{x} = x
\]
Square roots have several key properties and characteristics:
- Non-negative Result: The square root of a positive number is always non-negative. For example, \(\sqrt{25} = 5\), since \(5 \times 5 = 25\).
- Perfect Squares: If the number is a perfect square (e.g., 1, 4, 9, 16), its square root is an integer. For instance, \(\sqrt{16} = 4\).
- Irrational Numbers: If the number is not a perfect square, its square root is an irrational number, meaning it cannot be expressed as a simple fraction. For example, \(\sqrt{10} \approx 3.16227766016838\).
Square roots are applicable in various mathematical contexts:
- Geometry: Used to determine lengths of sides in right triangles and other geometric shapes.
- Algebra: In solving quadratic equations and other algebraic expressions.
- Physics and Engineering: Applied in formulas involving distance, speed, and other physical quantities.
Understanding square roots is crucial for grasping more advanced mathematical concepts. For example, the expression \(2 \sqrt{10}\) involves multiplying the square root of 10 by 2, highlighting the combination of basic arithmetic and square root operations.
Mathematical Representation of 2 Square Root of 10
The mathematical expression \(2 \sqrt{10}\) represents the product of the number 2 and the square root of 10. This can be broken down into a few simple steps to understand and calculate the value.
Firstly, the square root operation is defined as:
\[
\sqrt{x} = y \quad \text{if and only if} \quad y^2 = x
\]
For the number 10, its square root is expressed as \(\sqrt{10}\), which is an irrational number approximately equal to 3.16227766016838. This means:
\[
\sqrt{10} \approx 3.16227766016838
\]
Next, we multiply this value by 2. The multiplication operation can be represented as:
\[
2 \times \sqrt{10}
\]
Performing this multiplication yields:
\[
2 \times 3.16227766016838 \approx 6.32455532033676
\]
Thus, the value of \(2 \sqrt{10}\) is approximately 6.32455532033676. This value combines the properties of both multiplication and square roots, resulting in an expression that is widely used in various mathematical and applied contexts.
In summary, the representation of \(2 \sqrt{10}\) involves:
- Calculating the square root of 10, which is \(\sqrt{10} \approx 3.16227766016838\).
- Multiplying this value by 2, resulting in \(2 \sqrt{10} \approx 6.32455532033676\).
This expression is a useful mathematical construct that demonstrates the combination of basic arithmetic operations with irrational numbers, providing a foundation for more complex calculations and applications.
Decimal Approximation
The expression \(2 \sqrt{10}\) involves an irrational number, meaning its decimal representation is non-terminating and non-repeating. However, for practical purposes, we often use a decimal approximation to represent such numbers. Let's explore the decimal approximation of \(2 \sqrt{10}\) in detail.
-
Calculate the Square Root of 10:
The square root of 10 is an irrational number, which can be approximated as follows:
\[
\sqrt{10} \approx 3.16227766016838
\] -
Multiply by 2:
To find the value of \(2 \sqrt{10}\), multiply the approximate value of \(\sqrt{10}\) by 2:
\[
2 \times \sqrt{10} \approx 2 \times 3.16227766016838
\] -
Perform the Multiplication:
Carrying out the multiplication gives us:
\[
2 \times 3.16227766016838 \approx 6.32455532033676
\]
Thus, the decimal approximation of \(2 \sqrt{10}\) is approximately 6.32455532033676. It's important to note that this value can be rounded to various degrees of precision depending on the requirements of the specific application. For instance:
- To 2 decimal places: 6.32
- To 3 decimal places: 6.325
- To 4 decimal places: 6.3246
This approximation allows us to use \(2 \sqrt{10}\) in practical calculations where an exact irrational number would be cumbersome. Decimal approximations are widely used in fields such as engineering, physics, and computer science to simplify complex calculations while maintaining a high degree of accuracy.

Properties of 2 Square Root of 10
The expression \(2 \sqrt{10}\) has several interesting mathematical properties that make it significant in various contexts. Let's explore these properties in detail.
-
Irrational Number: Since \(\sqrt{10}\) is an irrational number, \(2 \sqrt{10}\) is also irrational. It cannot be expressed as a simple fraction, and its decimal representation is non-terminating and non-repeating.
-
Real Number: \(2 \sqrt{10}\) is a real number, as it represents a point on the number line. Both 2 and \(\sqrt{10}\) are real numbers, and their product is also real.
-
Positive Value: The value of \(2 \sqrt{10}\) is positive because both 2 and \(\sqrt{10}\) are positive. Therefore, their product is also positive.
-
Scale Factor: The multiplication by 2 acts as a scale factor, doubling the value of \(\sqrt{10}\). This property is useful in various scaling transformations in geometry and physics.
-
Approximation: The approximate value of \(2 \sqrt{10}\) is 6.32455532033676. This approximation is useful for practical calculations where exact irrational values are difficult to use.
Additionally, here are some mathematical operations and their properties involving \(2 \sqrt{10}\):
| Operation | Result | Property |
|---|---|---|
| Addition: \(2 \sqrt{10} + 2 \sqrt{10}\) | \(4 \sqrt{10}\) | Sum of two same irrational numbers |
| Subtraction: \(2 \sqrt{10} - \sqrt{10}\) | \(\sqrt{10}\) | Difference of multiples of irrational number |
| Multiplication: \(2 \sqrt{10} \times 2\) | \(4 \sqrt{10}\) | Product of a real number and irrational number |
| Division: \(2 \sqrt{10} \div 2\) | \(\sqrt{10}\) | Quotient of the product and the multiplier |
Understanding these properties of \(2 \sqrt{10}\) is crucial for its application in solving complex mathematical problems and in various fields such as physics, engineering, and computer science. These properties highlight the versatility and importance of the expression in mathematical operations.
Applications in Geometry
The square root of 10 can be applied in various geometric contexts, particularly in calculations involving lengths, areas, and volumes. Here are some specific applications:
- Pythagorean Theorem: In a right triangle with legs of length 2 and √10, the hypotenuse can be calculated as √(2^2 + (√10)^2).
- Area of a Rectangle: If one side of a rectangle measures 2 units and the other side measures √10 units, the area can be found by multiplying these two dimensions.
- Volume of a Cube: For a cube where each edge length is √10, the volume V is given by V = (√10)^3.
- Ratio of Circumference to Diameter: The value of 2√10 is related to the circumference-to-diameter ratio (π) of a circle with radius √10/2.
Applications in Physics
The value of 2√10 finds several applications in physics, particularly in calculations involving energy, waveforms, and fundamental constants. Here are some specific applications:
- Waveform Analysis: In wave theory, the amplitude of a waveform can be represented using √10, and consequently, 2√10 helps in analyzing wave properties such as intensity and frequency.
- Energy Calculations: Energy levels in certain quantum systems can be described using multiples of √10, influencing calculations of energy states and transitions.
- Atomic Physics: Constants related to atomic structures and interactions, such as the Bohr radius or the fine-structure constant, incorporate the value 2√10 in their derivations.
- Particle Physics: In particle accelerators and quantum field theory, values involving √10 contribute to formulas describing particle behavior, cross-sections, and decay rates.
Applications in Engineering
The value 2√10 is utilized in various engineering disciplines to solve practical problems and design efficient systems. Here are some notable applications:
- Structural Analysis: Engineers use √10 in calculations related to structural stability, load-bearing capacities, and material strength assessments.
- Electrical Engineering: In circuits and signal processing, 2√10 appears in calculations involving impedance, voltage levels, and signal amplification.
- Mechanical Engineering: In mechanical systems, √10 plays a role in determining forces, stresses, and dynamic responses in components such as gears and shafts.
- Fluid Dynamics: Engineers apply the value 2√10 to analyze fluid flow rates, pressure distributions, and turbulence in pipes and channels.
- Control Systems: In designing control systems for automation and robotics, √10 is used in equations that describe stability margins and response times.

Comparison with Other Square Roots
Comparing 2√10 with other square roots reveals interesting relationships and applications in various contexts:
- 2√10 vs. √2: While both are irrational numbers, 2√10 is approximately 4.472, whereas √2 is approximately 1.414. This difference influences their roles in geometry, physics, and engineering.
- 2√10 vs. √5: √5 is approximately 2.236, highlighting a significant contrast in numerical value and practical applications in mathematical calculations.
- 2√10 vs. √3: With √3 approximately equal to 1.732, the comparison showcases how 2√10 is a larger value, impacting areas such as trigonometry and geometric computations.
- 2√10 vs. √6: √6 is approximately 2.449, illustrating a different magnitude and utility in mathematical formulas and scientific analyses compared to 2√10.
Visual Representation
The visual representation of 2√10 helps in understanding its geometric and mathematical significance through graphical illustrations and diagrams:
- Number Line: Placing 2√10 on a number line between the integers 4 and 5 shows its position as an irrational number approximately equal to 4.472.
- Geometric Shapes: Visualizing 2√10 in geometric shapes such as rectangles, right triangles, and circles helps in demonstrating its application in area, perimeter, and other geometrical calculations.
- Graphs and Charts: Representing functions involving 2√10 on Cartesian coordinate systems provides insights into its behavior and relationship with other variables or constants.
- Comparative Visuals: Comparing 2√10 visually with other square roots, such as √2, √5, √3, and √6, highlights differences in magnitude and application across different mathematical and scientific contexts.
Common Misconceptions
Despite its mathematical clarity, 2√10 may lead to several common misconceptions:
- Confusion with Whole Numbers: Due to its non-integer nature, there might be confusion in understanding 2√10 as it falls between whole numbers 4 and 5.
- Complexity in Calculation: Some may perceive calculations involving 2√10 as complex or difficult compared to simpler arithmetic involving integers or well-known fractions.
- Application Limitations: Misconceptions may arise regarding the practical application of 2√10 in everyday scenarios, leading to underestimation of its utility in advanced mathematical and scientific calculations.
- Comparison with Integer Roots: Comparing 2√10 with integer square roots like √4 or √9 might lead to misconceptions about its magnitude and numerical properties.
FAQs
Here are some frequently asked questions about 2√10:
- What is 2√10?
2√10 is a mathematical expression representing the product of 2 and the square root of 10. - Is 2√10 an integer?
No, 2√10 is not an integer. It is an irrational number approximately equal to 4.472. - What are the applications of 2√10?
2√10 finds applications in various fields such as geometry, physics, engineering, and mathematical calculations where precise numerical values are required. - How is 2√10 used in real-world scenarios?
Engineers and scientists use 2√10 in structural analysis, waveforms, energy calculations, and other advanced mathematical models to solve complex problems and design efficient systems. - What are some misconceptions about 2√10?
Common misconceptions include confusion with whole numbers, complexity in calculations, and underestimation of its practical applications in everyday scenarios.

READ MORE:
Xem video hướng dẫn cách tính căn bậc hai của biểu thức (7 + 2√10) để giải quyết các bài toán liên quan đến từ khóa '2 căn bậc hai của 10'.
Hướng dẫn cách tìm căn bậc hai của (7 + 2√10)